CryptoDB
Coupling of Random Systems
| Authors: | |
|---|---|
| Download: | |
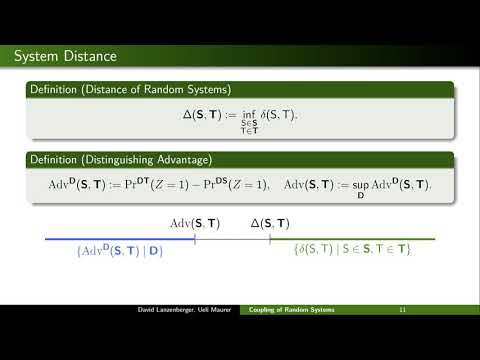
| Abstract: | This paper makes three contributions. First, we present a simple theory of random systems. The main idea is to think of a probabilistic system as an equivalence class of distributions over deterministic systems. Second, we demonstrate how in this new theory, the optimal information-theoretic distinguishing advantage between two systems can be characterized merely in terms of the statistical distance of probability distributions, providing a more elementary understanding of the distance of systems. In particular, two systems that are epsilon-close in terms of the best distinguishing advantage can be understood as being equal with probability 1-epsilon, a property that holds statically, without even considering a distinguisher, let alone its interaction with the systems. Finally, we exploit this new characterization of the distinguishing advantage to prove that any threshold combiner is an amplifier for indistinguishability in the information-theoretic setting, generalizing and simplifying results from Maurer, Pietrzak, and Renner (CRYPTO 2007). |
Video from TCC 2020
BibTeX
@article{tcc-2020-30599,
title={Coupling of Random Systems},
booktitle={Theory of Cryptography},
publisher={Springer},
author={David Lanzenberger and Ueli Maurer},
year=2020
}