CryptoDB
On Treewidth, Separators and Yao’s Garbling
| Authors: | |
|---|---|
| Download: | |
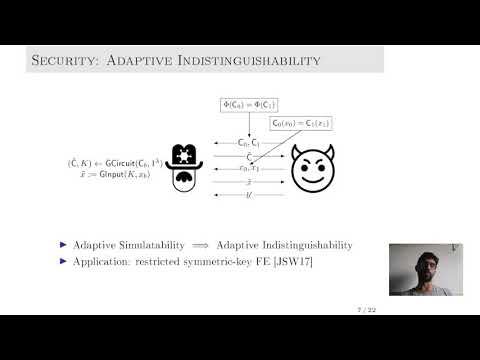
| Abstract: | We show that Yao’s garbling scheme is adaptively indistinguishable for the class of Boolean circuits of size S and treewidth w with only a S^{O(w)} loss in security. For instance, circuits with constant treewidth are as a result adaptively indistinguishable with only a polynomial loss. This (partially) complements a negative result of Applebaum et al. (Crypto 2013), which showed (assuming one-way functions) that Yao’s garbling scheme cannot be adaptively simulatable. As main technical contributions, we introduce a new pebble game that abstracts out our security reduction and then present a pebbling strategy for this game where the number of pebbles used is roughly O(\delta w log(S)), \delta being the fan-out of the circuit. The design of the strategy relies on separators, a graph-theoretic notion with connections to circuit complexity. |
Video from TCC 2021
BibTeX
@article{tcc-2021-31523,
title={On Treewidth, Separators and Yao’s Garbling},
booktitle={Theory of Cryptography;19th International Conference},
publisher={Springer},
doi={10.1007/978-3-030-90453-1_17},
author={Chethan Kamath and Karen Klein and Krzysztof Pietrzak},
year=2021
}