CryptoDB
Two-Round Oblivious Linear Evaluation from Learning with Errors
| Authors: |
|
|---|---|
| Download: | |
| Conference: | PKC 2022 |
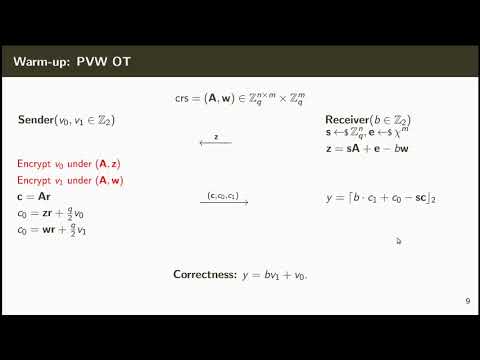
| Abstract: | Oblivious Linear Evaluation (OLE) is the arithmetic analogue of the well-know oblivious transfer primitive. It allows a sender, holding an affine function $f(x)=a+bx$ over a finite field or ring, to let a receiver learn $f(w)$ for a $w$ of the receiver's choice. In terms of security, the sender remains oblivious of the receiver's input $w$, whereas the receiver learns nothing beyond $f(w)$ about $f$. In recent years, OLE has emerged as an essential building block to construct efficient, reusable and maliciously-secure two-party computation. In this work, we present efficient two-round protocols for OLE over large fields based on the Learning with Errors (LWE) assumption, providing a full arithmetic generalization of the oblivious transfer protocol of Peikert, Vaikuntanathan and Waters (CRYPTO 2008). At the technical core of our work is a novel extraction technique which allows to determine if a non-trivial multiple of some vector is close to a $q$-ary lattice. |
Video from PKC 2022
BibTeX
@inproceedings{pkc-2022-31736,
title={Two-Round Oblivious Linear Evaluation from Learning with Errors},
publisher={Springer-Verlag},
author={Pedro Branco and Nico Döttling and Paulo Mateus},
year=2022
}